Understanding Option Greeks: Delta, Theta, Gamma, and Vega

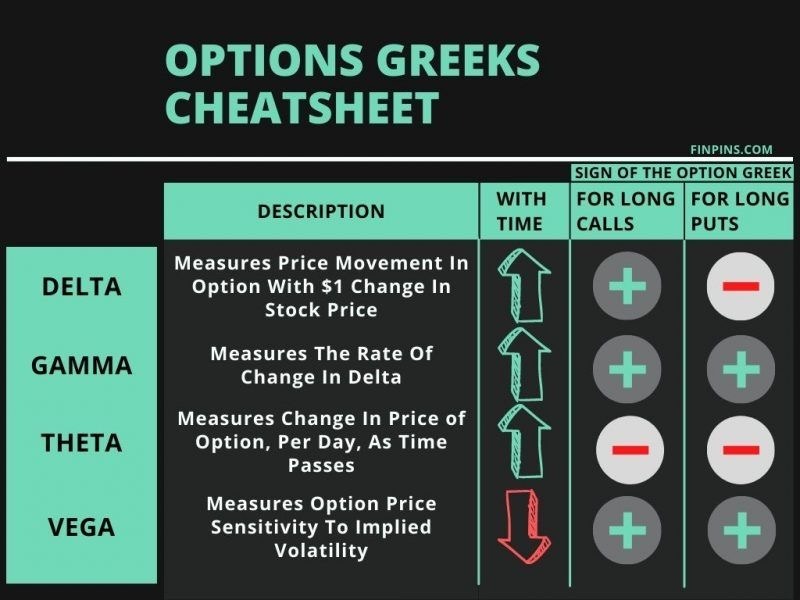
How to Use the Greeks in Trading Strategies

Understanding the Greeks—Delta, Theta, Gamma, and Vega—is essential for traders looking to optimize their options trading strategies. Each Greek provides critical insight into different aspects of options pricing and risk management. By effectively utilizing these metrics, traders can enhance their decision-making process and better manage potential losses.

Delta, for example, indicates the degree to which an option's price is expected to move in relation to a $1 change in the underlying asset. Traders often use Delta to construct hedged positions. For instance, if a trader holds a long position in an asset, they may buy a put option with a Delta value that offsets a portion of the underlying's risk, thereby reducing their exposure to price fluctuations.

Theta is associated with time decay, reflecting how the passing of time can erode an option's extrinsic value. Traders engaging in strategies like calendar spreads can profit from Theta decay. By simultaneously holding short-term and long-term options with the same strike price, traders can benefit from the rapid time decay of the short position while maintaining exposure through the long position.

Gamma measures the rate of change of Delta concerning movements in the underlying asset's price. This metric is particularly useful for dynamic hedging. For instance, a trader may adjust their Delta hedge frequently based on gamma readings to maintain a balanced risk profile as market conditions fluctuate.

Finally, Vega assesses sensitivity to volatility. Traders may employ straddles or strangles to capitalize on significant price fluctuations when expected volatility increases. By holding both call and put options at the same strike price, traders can benefit from unpredictable market movements, making careful consideration of Vega crucial.

Through these practical applications of the Greeks, traders can develop comprehensive strategies that enhance profitability while effectively managing risk. Understanding these metrics is pivotal for navigating the complexities of the options market successfully.

What is Delta?

Delta is a critical concept in the realm of options trading, serving as a key measure of an option's price sensitivity relative to changes in the price of its underlying asset. Specifically, Delta quantifies how much the price of an option is expected to change when the underlying asset experiences a $1 shift. For traders, understanding Delta is essential for assessing both directional risk and potential profitability of options trades.

Delta values range from 0 to 1 for call options and from 0 to -1 for put options. In the case of a call option, a Delta of 0.5 suggests that for every dollar increase in the price of the underlying asset, the call option's price is expected to rise by 50 cents. Conversely, a put option with a Delta of -0.5 indicates that a one-dollar increase in the underlying asset price would lead to a 50-cent decrease in the put option's price. This sensitivity to price changes makes Delta a valuable tool in formulating trading strategies.

Traders often utilize Delta to gauge their exposure to market movements. A high Delta value indicates that the option is more sensitive to price changes, making it beneficial for those seeking to capitalize on price movements. Conversely, a low Delta may suggest limited risk exposure but also reduced potential profit. For instance, deep in-the-money options typically exhibit a Delta nearing 1 (for calls) or -1 (for puts), while out-of-the-money options have a Delta closer to 0.

In summary, Delta serves as an essential metric in options trading, allowing traders to evaluate the potential impact of price fluctuations in the underlying asset on an option's pricing. By understanding and analyzing Delta, traders can better position themselves to make informed decisions and manage risk effectively.

Theta: The Time Decay of Options

Theta is a crucial component in options trading, representing the rate at which an option's price diminishes as it nears its expiration date. This phenomenon, commonly referred to as time decay, indicates that as time passes, the extrinsic value of an option erodes. Theta is particularly significant for traders, as it directly affects their trading strategies, especially for those engaged in selling options.

To elaborate, every option contract possesses a specific amount of time value, which decreases as the expiration date approaches. This decline is quantified by Theta, expressed as a negative number, indicating the amount by which an option's price is expected to decline for each passing day. For example, if an option has a Theta of -0.05, it implies that the option's price will decrease by approximately $0.05 daily, assuming all other variables remain constant. Consequently, options sellers often find themselves at an advantage during periods of significant time decay, as they collect premiums that decrease in value over time.

It is essential to note that Theta's impact is not uniform across all options. For instance, deep in-the-money options exhibit lower Theta values compared to at-the-money options, which tend to experience the greatest time decay as expiration approaches. As a result, traders need to consider their option's strike price and time until expiration when assessing potential risks and rewards. Additionally, different market conditions can influence Theta. In volatile markets, options may exhibit heightened pricing, affecting time decay in unpredictable ways.

Implementing a strategy that accounts for Theta can empower traders to optimize their positions. For example, in a stable market, an options seller might initiate a short position on at-the-money calls or puts. As the expiration date draws nearer, the time decay factor increases, potentially maximizing gains from the initial premium placed. Properly harnessing the effects of Theta can thus prove beneficial for traders looking to enhance their overall trading efficacy.

Gamma: Measuring Delta's Sensitivity

Gamma is a vital component in the realm of options trading, as it quantifies the rate of change of an option's Delta in connection with the price movements of the underlying asset. In simpler terms, while Delta indicates how much an option's price might change with a $1 change in the underlying asset, Gamma reveals how much Delta itself is likely to fluctuate as the asset price varies. This relationship is crucial for traders as it helps them understand the stability of their positions in the face of market volatility.

Traders often monitor Gamma closely, particularly during periods of heightened market uncertainty. A high Gamma value signals that Delta can change rapidly, which means the option’s price can also experience significant shifts. This sensitivity can lead to challenges in managing option portfolios, as traders must adjust their strategies in real-time to account for these fluctuations. For instance, if a trader holds a long position in an option with a high Gamma, even a small movement in the underlying price can result in a substantial change in Delta, necessitating potential adjustments to hedge the position effectively.

To illustrate, consider an example where an option originally has a Delta of 0.50 and a Gamma of 0.10. If the underlying asset moves up by $1, the Delta may increase to 0.60. However, if the underlying continues to rise, reaching $2 above the initial level, the Gamma effect becomes more pronounced, pushing the Delta closer to 0.80. This demonstrates that as the underlying asset fluctuates, the Gamma significantly impacts the Delta, underscoring its importance for traders in maintaining responsive and agile positions.

Understanding Gamma is essential for options traders, especially when navigating the complexities of fast-paced market shifts. By incorporating Gamma analysis into their trading strategies, they can better anticipate Delta's movements and adjust their exposure, thereby enhancing overall portfolio management.

Vega: Sensitivity to Volatility

Vega is a crucial Greek in options trading, representing the sensitivity of an option's price to changes in the volatility of the underlying asset. It measures how much an option's price is expected to change for a one-percentage point increase in implied volatility. In this context, it is important to understand that volatility often reflects the market's expectation of future price fluctuations, which can significantly impact option pricing.

When volatility increases, option prices typically rise, as the potential for price movement enhances the likelihood of options being profitable at expiration. Conversely, when volatility declines, option prices generally decrease. This relationship underscores the importance of managing Vega within options trading strategies. For instance, traders may employ straddles or strangles, which involve buying both call and put options on the same underlying asset. Such strategies benefit from increased volatility, potentially leading to higher profits if the underlying asset makes a significant move in either direction.

On the other hand, if a trader anticipates a decrease in volatility, they may choose to sell options, as the resulting decline in time value can increase profitability. Similarly, options traders often assess Vega in conjunction with other Greeks to develop comprehensive strategies. For example, knowing the Vega of a position allows traders to gauge how sensitive their portfolio is to volatility changes, enabling them to make more informed decisions.

Moreover, different options exhibit varying levels of Vega, largely influenced by their expiration dates and the underlying asset's characteristics. In-the-money options, for example, tend to have lower Vega compared to out-of-the-money options, which are more susceptible to volatility changes. Understanding these nuances empowers traders to navigate the complexities of Vega and utilize it effectively in their trading strategies.